QUIZ 3
READ ALL EXAMPLES. SOME PROBLEMS ARE JUST LIKE AN EXAMPLE. 
CH. 2 & 3 
CH. 2 - Multiple choice 9, 10, 14, 15  and exercise/problems FREE FALL (READ EXAMPLES 2.10 AND  2.11  !)  *48, *51, *53, *56, *59, *60, 73*, 75*, 79*, 83*; RELATIVE MOTION 61*, 64* (See example 2.12 !)  
CH. 3 - INTRODUCTION TO PROJECTILE MOTION (MORE SUCH PROBLEMS ASSIGNED IN QUIZ 4 COMING UP) Multiple choice 1, 2,  3, 4, 5, 6  (note- 4 and 5 are  1D questions exploring the y-motion occurring in 2-D projectile motion) exercises/problems 12*, 18*, 56* . 
* DISCUSSIONS PROVIDED. 
SELECTED DISCUSSIONS TO exercises/problems BELOW.
ALL PROBLEMS ARE DUE EVEN THE ONES WITHOUT DISCUSSIONS, WHICH ARE DESIGNED TO HELP YOU DO RELATED EXERCISES.
For problems below, unless otherwise stated, assume up is positive, and thus the  downward directed acceleration component is negative: ay = -g.
48. (a) Use V2y2 = V1y2 - 2*g*(change in y) and set the second velocity, at the top, equal to zero. Find the first velocity.
(b) Find the total  time  by using
change in y = V1y *t   - (1/2)*g*t2.
Set change y = 0 = V1y *t   - (1/2)*g*t2  = t*[ V1y    -  (1/2)*g*t ] and solve  V1y    -  (1/2)*g*t  = 0 for the total time.

Note you can also get the total  time by getting the time  to arrive at the top of the path by  using V2y = V1y    - g*t  and setting the second velocity = 0 and solving for t. The total time of flight is twice this value !!
(c) the magnitude and direction of the acceleration are both constant ( do not change) over the entire motion .
Below is another way of seeing the problem:

Set Up:  Let  be upward.    at the maximum height.

Solve:  (a)        gives

(b) When the flea returns to ground,    gives

(c)  downward, at all points in the motion
51. (a) The total time is 8.5 seconds, so the time to get to the top (where the velocity is zero) is 4.25 seconds. You can find the initial velocity using :
V2y  = 0 = V1y    - 0.379g*t   and plugging t = 4.25 seconds. Find V1y . Then use V2y2 = 0 =  V1y2 - 2*0.379g*(change in y) and find change in y. 
(b) see part (a)
(c) See figure 2.28. The section  of the graph you are interested in is between 0 and 3 seconds. In the current  problem you replace 3 seconds by 8.5 seconds and g by 0.379g.
53. (a)  Use  V2y  = V1y    -   g*t  and    change in y = V1y *t   - (1/2)*g*t2  for the two times given.  Remember to add
40.0 m to change in y. Note the first velocity is +5.00 m/s.
(b) Use change in y = - 40.0 m  =  V1y *t   - (1/2)*g*t2.  and solve the quadratic  equation for t.  Note the first velocity is +5.00 m/s.
(c) Use V2y  = V1y    -   g*t and the time t from the previous part. You can also use
V2y2 = V1y2 - 2*g*(change in y), where change in y = - 40.0 m, in order to get the second velocity.  Note take the negative square root. The first velocity is +5.00 m/s.
(d)    Use V2y2 = V1y2 - 2*g*(change in y) and set the second velocity, at the top, equal to zero. Find change in y. Remember to add 40.0 m to your answer.
(e) See figure 2.28 as a guide.
56. (a) Use  V2y  = V1y    -   g*t . Note the initial ( first) velocity is negative even though the speed is given: 
V1y= - 15.0 m/s.

(b)  change in y = V1y *t   - (1/2)*g*t2.  Note the initial ( first) velocity is negative.  
(c) Use V2y2 = V1y2 - 2*g*(change in y).  Note: change in y = -10.0 m. When you solve for the speed |V2y |, take the positive square root since you are finding the speed. If you wanted the second velocity V2y  you would take the negative square root, since down is negative.
Below is another way of seeing the problem:

Set Up:  Take  downward.

Solve:  (a)

(b)

(c)  gives


59. (a) From #48, we know the total time of flight = 2* V1y  / g, where V1y is the initial velocity.
Solve for the initial velocity of the faster stone and then the initial velocity of slower stone. Then use
time of flight = 2* V1y  / g again to get the time of flight of the slower stone. 

(b) Use  V2y2 = V1y2 - 2*g*(change in y) and set the second velocity, at the top, equal to zero. Note if we are dealing with the slower stone, the change in y = H. Solve for the initial velocity of the slow stone in terms of H. Then find the initial velocity of the faster stone in terms of H by using  the relationship between the initial velocity of the fast stone and the initial velocity of the slow stone.

60. In this problem you will encounter the square root of 2. 
(a)  Let the first coconut (A)  be dropped from H and the second coconut  (B) dropped from H/2.
 VA2  = 2gH  and VB2 = 2g(H/2)  are the squares of the speeds when each hits the ground. Take the ratio, then square root to get the relationship between the two speeds at impact with ground.  Note |VA  | = V.  Write the B coconut speed in terms of V. 
(b) |VB | = gT and |VA | = g*time.  Find the time of the A coconut in terms of T using the relationships you found in part (a). Your answer will involve a square root of 2. 
Below is another way of seeing the problem:

Set Up:  Take  to be downward and  Both coconuts have the same acceleration,  Let A be the coconut that falls from the greater height and let B be the other coconut.  

Solve:  (a)  gives  and  

(b)  gives  and  


73. SOLVE A VERTICAL  FREE FALL  EQUATION FOR TIME OF FLIGHT OF EGG. THEN SUBSTITUTE INTO PROFESSOR'S UNIFORM HORIZONTAL MOTION EQUATION.
In particular, we can set the downward to be the positive y direction. In this case, 
change in y = V1y *t + (1/2)*gt2.  Since the egg is dropped, V 1y   = 0.   Solve for the time t.  Note the change in y is positive but is less than 46.0 m since the egg hits the head of a 1.80 m tall professor.  Thus you must subtract 1.8 m from 46 m to get change in y. The plug time t into the formula for the horizontal displacement of the professor:  x = V*t, where V is the horizontally directed l speed of professor as she moves to the right.  
75.   (a) Use V2y = V1y - gt and find the  the requested time t given V2y = + 20 m/s.  
(b)  Repeat for  V2y = - 20 m/s    . 
(c) V2y = - 40 m/s 
(d) V2y = V1y - gt . Set the second velocity equal to ZERO and find t.  
(e)  See figure 2.28. The section  of the graph you are interested in is between 0 and 3 seconds.
79. To begin this problem find the height  and velocity the rocket goes before shutting off the engine.
change in y =  V1y *t  + (1/2)*(2.50 m/s2)*t2.  and  V2y = V1y  + (2.50 m/s2 ) t,   where the first velocity is ZERO since the rocket begins from rest. t = 20.0 seconds. 
(a)    Use VBy2 = VAy2 - 2*g*(change in y). Set  the speed at point B, the highest point, equal to ZERO. Find the change in y and add  it to the  value of change of y you got when the engine was on.  Note: The VAy =  V2y   you found above.
(b) At the highest point the velocity is zero but the acceleration is not zero. 
(c) The time can be found in two   pieces: 1. while the engine is on and 2. while it rocket is rising and falling in free fall 
 (engine shut off) .
1. The first time  is  20.0 seconds
2.  You know the total change in y from shut off to when it hits the ground.  It equals the negative of the  vertical displacement between launch and shut off.  You calculated the vertical displacement between launch and shut off at the beginning the problem above: change in y =   (1/2)*(2.50 m/s2)*t2, where t = 20.0 s.  Solve the following quadratic equation for t', the time the rocket is in free fall.    -change in y =  V2y  *t'  - (1/2)gt'2  . 
The total time is 20.0 s + t'.  
 You can get the velocity when it hits the ground this way: V3y =  V2y    - gt' .
There are other ways to solve this problem without a quadratic equation  which will be discussed in class.
83. Let t1 = time to hit the bottom of cliff and t2 = time the sound takes to get from bottom of cliff to your ear at the top.
Let H be the unknown cliff height. We know H = (1/2)*g*t12.  Also  t2 = H/(330 m/s) and  t1 = 10.0 s -  t2  .   Substitute and  solve for H = (1/2)*g*( 10.0  - H/330)2.  Multiply out the right hand side and solve for H. Solve a  quadratic equation for H.  Clearly if you neglected the speed of sound, you would have overestimated the cliff height. 
61. See example 2.12
64. Hint: If no wind is blowing,  the trip is simply 2*L/V, where L = 2,000 mi and V = 600 mi/h.  If the wind is blowing west to east, relative to the ground the plane has larger speed than on the way to Chicago,  and relative to the ground the plane has a smaller speed from Chicago to SF. Thus on the way to Chicago, the plane takes less time and on the way back the plane takes more time.   
Below is another way of seeing the problem:

Set Up:    and is east for the first 200 mi and west for the return 200 mi. The time is the distance relative to the ground divided by the speed relative to the ground.

Solve:  

(a)  and  

(b) San Francisco to Chicago :  east.  east.

 

Chicago to San Francisco :  east.  west.

 

The total time is


CH. 3 Intro to Projectile Motion
USE EQUATIONS 3.12, 3.13, 3.14 AND 3.15 PAGE 77. AND SEE FIGURE 3.11 WHICH WE REVIEWED IN CLASS.
We assume up is the positive direction, such that the acceleration is straight down and has component ay = -g. THERE IS NO ACCELERATION IN THE X DIRECTION. THAT IS WHY THE X-COMPONENT OF VELOCITY IS CONSTANT.
12.  See example 3.3.  THIS JUST LIKE THE SIMULATION LAB. Referring to the equations mentioned above, we see  for the x-motion, 
x = Vo*cos0*t = Vo*t since the launch angle is zero. Thus Vx = Vo.
Y-MOTION:
y = -(1/2)g*t2 since sin0 = 0.
Vy =  -g*t since sin0 = 0. 

y = -9.00 m = -(1/2)*gt2. Solve for t.
x =1.75 m = Vo*t.  Solve for Vo.
Below is another way of seeing the problem:

Take +y downward.  She must travel 1.75 m horizontally during the time she falls 9.00 m vertically.

Solve:  Time to fall 9.00 m:  gives


Speed needed to travel 1.75 m horizontally during this time:  gives





18. This is admittedly a sophisticated problem. It may be helpful to do #56 first, which simulates figure 3.11.
For the stone, the initial velocity components are:
Vox = Vo*cos theta = 15 m/s
Voy = -Vo sin theta = - 20 m/s. Note the y-component of velocity is negative.
Also note we do not need the initial  launch angle theta in order to find the requested info.
X-MOTION:
x = (15 m/s)*t.
Y-MOTION
Vy =  -20 m/s  - g*t
and
y =    -20 m/s*t - (1/2)*g*t2.

(a) You are given the time t = 4.00 s. Plug this time  into the y-motion equation for the vertical displacement to get
 y =  -20 m/s*t - (1/2)*g*t2.  We are assuming y = 0 at the  initial level of the balloon. Take the absolute value of the answer to get the initial height H of the balloon: H = |y|
(b) x = (15 m/s)*t
(c) This is a little tricky. The distance of the rock from the balloon is given by the Pythagorean Theorem. You need to get the x displacement of the rock from the balloon first which is given by x = (15 m/s)*t,  where you plug in t = 4.0 seconds.
Now you need to get the y component of displacement of the rock from the balloon. That simply is the difference between the height you computed in part (a) and the vertical distance moved by the balloon in 4.0 seconds: 20.0 m/s*t - H.
The distance D between the rock and balloon is given by D2 = x2 + (20 m/s*t - H)2. Take the square root of both sides to get  D.      
Below is another way of seeing the problem:

Set Up:  Use coordinates with the origin at the initial position of the stone and +y downward.    The stone has  and

Solve:  (a) Find the vertical distance the rock travels in 4.00 s.

(b)

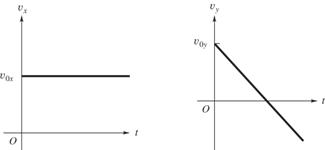
(c) The basket descends (20.0 m/s)(4.00 s) = 80.0 m in 4.00 s so is 158 m - 80.0 m = 78.0 m above the ground when the stone reaches the ground. The stone is a distance  from the basket when it hits the ground.


56. Study examples 3.4. and 3.5.  We will review this in class. Vx will be constant for the entire trip so the plot Vx vs t  will be a straight horizontal line (zero slope) . The plot of Vy vs t will look like that of figure 2.28 (b), where the slope = -g.
Below is another way of seeing the problem:

Set Up:  Use coordinates with +y upward.

   and

Solve:    since    The graphs of  versus t and  versus t are given in the figure below. The graph of  is a straight horizontal line and the graph of  is a straight line with slope  at the maximum height.